Help the (skinny) Hedgehogs | 31 Oct. 2019 |
For a long time this picture - as taken from Gabriel Peyré his outstanding twitter feed - has been on a wall in our local wildlife (inc. hedgehogs) shelter. This has lead to a few fun discussions and here we try to explain the picture a bit more.
Let 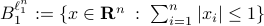 . Now, we want to find the largest ball
. Now, we want to find the largest ball 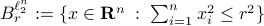 within
within  . We can find that
. We can find that 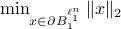 is given by
is given by  with
with 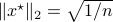 . This follows the most easily from geometric intuition.
. This follows the most easily from geometric intuition.
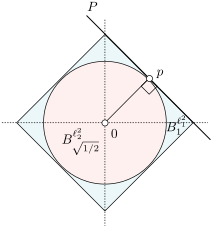 |
To prove it, see that |
Besides being a fun visualization of concentration, this picture means a bit more to me. For years I have been working at the Wildlife shelter in Delft and the moment has come where the lack of financial support from surrounding municipalities is becoming critical. As usual, this problem can be largely attributed to bureaucracy and the exorbitant amount of managers this world has. Nevertheless, the team in Delft and their colleagues around the country do a fantastic job in spreading the word (see Volkskrant, RTLnieuws). Lets hope Delft, and it neighbours, finally see value in protecting the local wildlife we still have.
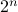 ‘‘similar’’ faces, without loss of generality we take the one in the positive orthant of
‘‘similar’’ faces, without loss of generality we take the one in the positive orthant of 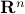 . Now we fit a
. Now we fit a 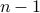 -dimensional plane
-dimensional plane 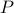 (hyperplane) to this face and find the point
(hyperplane) to this face and find the point 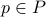 the closest (in
the closest (in 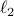 -norm) to
-norm) to 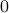 . This hyperplane can be parametrized by
. This hyperplane can be parametrized by 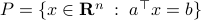 for
for 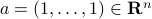 and
and 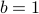 . Thus the normal (direction) is given by
. Thus the normal (direction) is given by  . To find
. To find  , observe that for
, observe that for 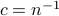 we have
we have  . Hence we have
. Hence we have 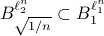 . Now, let
. Now, let  and define the other
and define the other 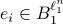 for any
for any 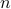 we can think of a (skinny) hedgehog indeed for
we can think of a (skinny) hedgehog indeed for  . The inscribed ball shrinks to
. The inscribed ball shrinks to